|
telescopeѲptics.net
▪
▪
▪
▪
▪▪▪▪
▪
▪
▪
▪
▪
▪
▪
▪
▪ CONTENTS
4.7.3.
Measuring chromatic error in an achromat: polychromatic PSF
Summing it up, an achromat optimized for a particular wavelength, will have spherical aberration canceled for
that wavelength, and chromatic aberration nearly cancelled
laterally, while reduced to nearly f/2000 of the F/C secondary
spectrum. The error at the optimum focus results from other wavelengths
being:
(1) defocused, and (2) affected by spherical aberration, with the
latter being comparatively low or negligible. The main error component,
that of chromatic defocus, can be expressed as a P-V wavefront error:
W = Pρ2
(51)
with P=-Δf/8F2 being the peak aberration coefficient, equal to the
P-V wavefront error,
and ρ
the pupil ray height in units of the pupil radius. This error combines
with the error of spherochromatism for that particular wavelength, and
the combined error is finally "measured up" by the
sensitivity factor of
the eye.
With
Δf
being, for the typical achromats, ~f/2000 at best, the P-V wavefront error
of chromatic defocus at its red and blue foci can be written as:
W ~ D/16,000F
(51.1)
For D=100mm and F=10, this gives 0.000625mm, or
1.29 wave P-V of defocus for the blue F-line (λ=0.000486mm), and 0.95
wave P-V of defocus for the red C-line (λ=0.000656mm).
For film/CCD applications, defocused
wavelengths are more to much more detrimental, depending on both,
characteristics of the chromatic defocus and spectral sensitivity of the
detector. For instance, most achromats have defocus error significantly
greater toward the blue/violet end, which would seriously impair
performance with a detector with high sensitivity for that range.
However, if the detector is relatively insensitive in the blue/violet, a
decent to good results can be achieved even with relatively fast
achromats, with significant gross chromatic errors.
Contrary to the common misconception,
nominal chromatic defocus in the
red and blue is not an accurate
indicator of the level of chromatism in an achromat, in the sense that the two change at a
different rate with the change in either aperture D or focal
ratio F. For instance, while the defocus error at any
wavelength other than the optimized changes either in proportion to the
change in aperture
D, or in inverse proportion to the change in focal ratio F, resulting chromatism - measured
as a change in defocus error corresponding to achromat's polychromatic peak diffraction intensity
(PPDI), or polychromatic Strehl (SP) -
changes at a significantly slower rate. For instance, an f/10
achromat has only half the secondary spectrum of an f/5,
but its polychromatic Strehl is only smaller by a factor of 21/3
(0.79 vs. 0.63 for 100mm aperture diameter, which corresponds to the RMS
wavefront error smaller by a factor of 0.51/2).
It further slows down for the longer-focus systems, with the Strehl for
a 100mm f/20 vs. f/10
achromat increasing by a factor of 21/5.
(0.90 vs. 0.79, corresponding to the RMS wavefront error reduction by a
factor of 0.50.6).
Since the RMS wavefront error is
proportional to the P-V error, the actual wavefront error for an
achromat of given aperture changes approximately in inverse proportion
to the square root of its focal ratio. Actual chromatic error in an
f/10 achromat is only 0.71 of that in an
f/5, but in the latter it is also only 1.4
times larger than in the former. This is what
the advanced optical design
software programs, using diffraction calculation, imply (note that
the value of PPDI in the visual range
doesn't change with scaling doublet achromat while keeping the
focal-ratio-to-aperture ratio F/D constant: 100mm f/12 has identical PPDI
as 200mm f/24).
The reason for this "strange" behavior is that much of defocused light
of the farther-off-optimal wavelengths is already out of the Airy
disc, and merely gets spread out wider (for instance, F and C line in the 4"
f/10 above have only a few percent of the energy left within the Airy
disc). The spectral range relatively close to the
optimized wavelength does not contribute significant "new" lost energy, since it
is relatively little affected. It is only a relatively narrow spectral segment on either side
of the optimized wavelength, which previously had small but appreciable
error that adds significant new energy to that
already transferred outside of the
Airy disc. A parallel can be drawn between any
far-from-focus wavelength, or a narrow spectral range, and a surface
error limited to a relatively small area. Such surface error keeps
draining more energy from the Airy disc with the increase in the nominal
error only up to the point when practically all the energy available
from that area is lost. After that, there is no appreciable effect from
the
further error increase. This is why turned edge
behaves as it does, or any wavefront error limited
to a relatively small area. For instance, a zone 1/10 of pupil radius
wide,
at half the radius, going from 1 to 2 waves P-V, won't change neither peak
intensity value (0.96) nor encircled energy (0.95),
despite the consequent doubling of the nominal (and at 0.22 and 0.44,
respectively rather substantial) RMS error. Heavily
defocused far wavelengths in an achromat are alike those
relatively
small in area, but nominally large and effect-wise mainly drained out wavefront errors.
This helps explain surprisingly good performance of fast
achromats in general - and particularly large fast achromats - which,
according to their nominal secondary spectrum, should be hardly usable
at all.
As it often goes in life, there is the bad side to it
as well: it is that the polychromatic Strehl also improves at a slower rate with the decrease in
nominal chromatic defocus; in other word, halving the focal ratio
doesn't halve the chromatism. The
good news is that the discrepancy between decrease in nominal defocus
error and the actual chromatic error is significantly smaller here.
While polychromatic Strehl is a reliable general
indicator of the effect of aberration over the range of resolvable
frequencies, it gives no information about more specific effects on
contrast transfer
within sub-ranges of frequencies that could be of interest. In
particular, how the effect of chromatism compares to the spherical
aberration effect at mid-to-low MTF frequencies (details approaching
Airy disc diameter, and larger; the range of planetary
and deep-sky observing) and near the stellar resolution threshold.
Differences in this respect can be expected based on distinctly
different form of energy distribution caused by chromatic error. Due to
the increasing defocus error for non-optimized wavelengths, diffraction
pattern has a form similar to one caused by monochromatic defocus: the
first dark ring is filled with energy, with its contrast deep
dramatically reduced, or non-existent.
As a result, at any given nominal peak intensity
(within the range commonly encountered with amateur telescopes),
diffraction pattern affected with secondary spectrum has higher encircled energy
fraction within the central maxima than what is indicated by the peak
value (Strehl). For instance, while the polychromatic e-line Strehl of a 100mm f/12 doublet achromat is 0.77,
and its best focus Strehl 0.81, the encircled energy within the Airy disc is 0.83. It is generally
higher than the encircled energy fraction with spherical aberration at
the same nominal peak intensity, which is nearly identical to the
encircled energy fraction. This effectively increases the relative
energy contained in central maxima vs. energy transferred to the rings
for given nominal Strehl, enhancing contrast transfer for extended
objects. On the flip side, the slight enlargement of central maxima due
to chromatic defocus does have negative
effect on the efficiency of contrast transfer details in that size range. The combined effect of
these two factors, as the MTF plots below illustrate, is
better contrast transfer at mid-to-low frequencies, and lower in the
0.5-0.8 frequency range (approximately).
Consequently, assessment of the optical quality of an achromat
varies somewhat with the focus location:
peak diffraction intensity (SP)
is not at the optimized (e-line) focus (SPe),
but closer to the d-line focus. And for any given Strehl, an achromat
will have better contrast transfer in the range of extended details than
an aperture with equal Strehl resulting from spherical aberration (and
most others), thus can be assigned a better effective Strehl (Sext).
Graphs below (FIG. 73)
and the accompanying text describe more specifically how the effect of
secondary spectrum in an achromat with standard glasses depends on its
aperture and focal ratio. Since its magnitude, with the given glasses,
is determined by these two parameters alone, it is not surprising that
it is a function of their combined form, F/D. As will be explained in
more details below, peak diffraction intensity in an achromat is not at
coinciding with its optimized wavelength's focus. Rather, it is shifted
somewhat toward the rest of wavelengths focusing behind it.
Gain in the Strehl and contrast transfer at the best focus
location is relatively small, but not negligible. Both,
Strehl values and MTF are calculated by OSLO,
based on 25 wavelengths from 440nm to 680nm (10nm increment), weighted
for the photopic eye sensitivity, for the standard
C-e-F Fraunhofer doublet achromat (BK7/F2, with
secondary spectrum
Δf~f/2000
with respect to d-line, and
Δf~f/1800
with respect to e-line). Note that the same polychromatic input is
used for calculating comparative effects of spherical
aberration.

FIGURE 73:
Effect of secondary spectrum on image quality in terms of
standard indicators of optical quality - central diffraction intensity
and contrast transfer efficacy - allows for its direct comparison with
other forms of aberrations, as well as a qualitative assessment of its
performance level. While the nominal chromatic defocus error for given
aperture D scales inversely with the focal ratio F, and
with the aperture for given focal ratio, the effective error - the one
that corresponds to the polychromatic Strehl, either at the best diffraction
focus, e-line focus, or for the "effective" Strehl - changes
approximately with the cube root of the square of either parameter. For
instance, chromatic defocus error for given aperture halves nominally
going from f/10 to f/20, but the effective error, corresponding to the
best focus Strehl, only reduces by 35%, from 0.275 to 0.18 wave P-V of
spherical aberration (it is nearly identical to wavefront error of
defocus, with the latter being larger by 3.3% for given RMS). An f/34
has the nominal chromatic defocus smaller by a factor of 0.3, but the
effective wavefront error (0.12) only by a factor of 0.44, and so on.
For short-focus achromats, additional
limiting factors is the emergence of higher order spherical aberration,
due to the strongly curved inner surfaces. This affects all the wavelengths,
and quickly brings down (the remaining) optical quality with further
focal ratio reduction. Small apertures are more limited by it in terms
of F/D ratio: at the ratio value of 1, for D in inches, the focal
ratio is still f/8 for an 8-incher, while only f/4 for 4-inch aperture.
There is no simple accurate expression for
achromat's polychromatic Strehl, but it
can be well approximated with simple empirical relations. Following text
addresses more specifically the three points of assessment of achromat's
optical quality:
(1) polychromatic Strehl at the optimized wavelength, (2) polychromatic
Strehl at the best focus location, and (3) effective polychromatic Strehl
for extended objects.
1 - Polychromatic Strehl at the
optimized line focus (SPe)
It is the peak diffraction intensity measured at the optimized
line (assumed e-line) focus in the standard Fraunhofer-type doublet achromat. as a function of
the aperture size D and
relative focal ratio F. Weighted for photopic eye sensitivity in 430-680nm
range, in the standard achromat with
the d-line secondary spectrum
Δf~f/2000,
it is
approximated by:
SPe ~ 1.3(F/Dmm)1/4
(a)
For D in inches,
SP~0.58(F/D") 1/4. It stays close to the actual value for F/Dmm
values smaller than ~0.25, which covers most practical instruments. For
larger values of F/Dmm it becomes too optimistic (it gives
SPe=1 for
F/Dmm=0.35, which corresponds to 100mm f/35, or 200mm f/70).
For (F/Dmm)
values greater than 0.2, up to ~0.8 (i.e. for very long focus achromats), better empirical approximation
for polychromatic Strehl in an achromat is
SPe~(F/Dmm)0.08,
or
SPe~0.8(F/D")0.07
for D in inches.
For F/Dmm
values of ~0.05 and smaller, the approximated polychromatic
Strehl gradually becomes too optimistic, mostly due to the overall deterioration
caused by increasing spherochromatism. Smaller apertures are more
affected, due to higher-order spherical aberration generated by their
steep inner radii. For instance, a 150mm
f/4.5 has polychromatic Strehl
of 0.50, while 100mm f/3 drops to 0.38 Strehl, despite both having
F/Dmm=0.03,
for which the above approximation gives 0.54 value.
The corresponding comparable RMS error of monochromatic aberration, obtained from
Eq. 56,
is RMS=0.24(-logSPe) 0.5.
2 - Peak polychromatic Strehl (SP)
However, polychromatic diffraction calculation also reveals a little
known - yet rather obvious - fact, that best polychromatic focus in an
achromat does not coincide with the focus of optimized wavelength
(usually around e-line). It is shifted somewhat toward the red/blue
focus, closer to the d-line focus. It is the consequence of all other
wavelengths focusing farther away than the optimized wavelength,
including those to which the eye is still highly sensitive. Up to a
point, reduction in defocus error in all the wavelengths away from
e-focus by shifting focus
location toward red/blue focus overcompensates for the increase in e-line
(and the immediate wavelengths) defocus. As a result, diffraction maxima
generates more energy.
I am not aware that this fact, stumbled upon accidentally while going
through matters related to Neil English's book about refractors, was
known at all; there is no mention of it by Sidgwick or Conrady
(understandably, since they did not have the benefit of accessible
diffraction calculation), nor more contemporary texts on aberrations,
including my cited references).
Graph bellow gives specifics of this effect for a standard
100mm f/10 achromat.

This implies that best polychromatic ratio in an achromat is higher than
what is indicated by (a), which approximates Strehl value at the
focus of optimized wavelength, usually around green e-line. As the inset "F-Ratio Dependence"
shows, the gain in polychromatic Strehl by refocusing from e-line to
best polychromatic focus - which is fair to assume that the eye leads us
to do - is slightly decreasing from fast toward slow systems. Again,
based on OSLO output, the highest polychromatic Strehl, as specified
earlier, is higher approximately by a factor of (F+7)/(F+6), F
being, as before, the focal ratio number.
Thus, peak polychromatic Strehl of an achromat is approximated by:
S P~ (F+7)Spe/(F+6)
(b)
with
Spe
being the polychromatic Strehl at the location of e-line focus,
as given by (a). Note that (a) applies only to F/D values
~0.2 and smaller; for F/D values larger than ~0.2, e-line polychromatic
Strehl is approximated by
Spe~(F/D)0.08
for D in mm, and by Spe~0.8(F/D)0.07
for D in inches. For F/Dmm
values of ~0.25 and higher, polychromatic Strehl at the best focus is
closely approximated by (F/D)0.06.
This strictly applies only to achromats
with negligible spherical aberration. That is fair assumption with
long-focus objectives, where fabrication tolerances are very wide, but
with mid and short focus achromats it is realistic to expect some degree
of correction error. In general, spherical aberration will reduce the
gain of refocusing. For instance, λ/4
P-V wavefront error of overcorrection will roughly cut it in half, also
reducing the extent of refocusing to a similar degree. On the other
hand, λ/4
of undercorrection while reducing the gain similarly, will have little
effect on the extent of refocusing.
(2): The effective Strehl for
extended details (Sext)
Another interesting
property of the achromat revealed by diffraction calculation
shows that the central
maxima consistently
encircles more energy than the same nominal Strehl of spherical
aberration. The significance of it is that the latter is the only other
"default" axial aberration, and even more that it is the dominant
aberration form in apochromats. Thus comparison by the nominal
polychromatic Strehl may not tell the whole story.
The effects is illustrated below with the
polychromatic diffraction PSF, encircled energy and MTF plots of a 100mm
f/6 achromat, whose 0.67 Strehl at the best polychromatic focus
nominally equals that of 1/3 wave P-V of primary spherical aberration.
While both have 0.67 nominal Strehl, the encircled energy within first
maxima is 0.63 for the achromat, and 0.58 for spherical aberration. The
energy nearly equalizes after the first bright ring, but energy
distribution up to that point favors the achromat, and it shows as
better MTF contrast transfer in the 0.15-0.5 frequency range. It is
compensated for with lower contrast transfer in the 0.5-0.7 frequency
range, but the lower range - approximately details 1-2 times the Airy
disc size - is more important for general observing, affecting the
bright low-contrast detail resolution threshold. For spherical
aberration to reach that level in this frequency sub-range, it would
need to be at about 0.28 wave P-V (0.76 Strehl), or to be "switched" to about 10%
larger aperture.
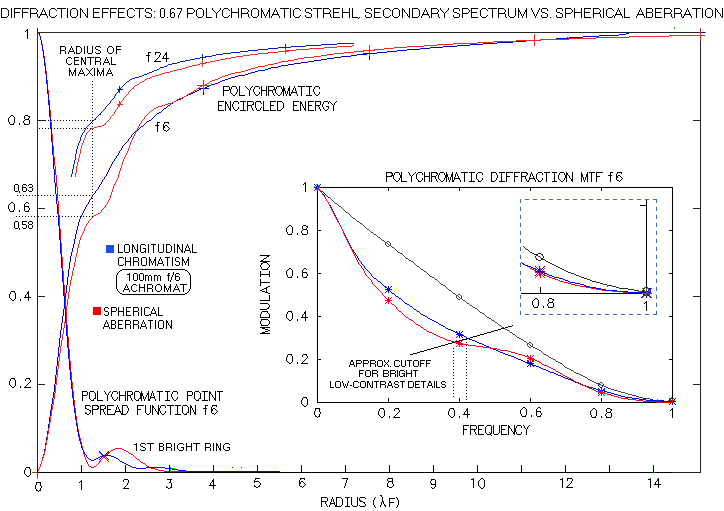
Expectedly, the difference diminishes with the magnitude of aberration.
Very approximately, the
ratio of
encircled energy for secondary spectrum vs. spherical aberration changes
in proportion to (F+7)/(F+6), F being, as before, the focal
ratio. The distribution profile is not the same, however, with the long
focus achromats apparently having more encircled energy not only within
the first dark ring area, but over a significantly wider radius as well.
There is no indications that either brightening of the
first dark ring caused by secondary spectrum, or intensifying and
enlarging the 1st bright ring by spherical aberration at these levels of
aberrations, lowers limiting stellar resolution - inasmuch as MTF graph
can show this resolution aspect. Considering that spherical aberration
and central diffraction obstruction generate similar diffraction effect,
it is fair to assume that secondary spectrum would have similar
advantage over central obstruction causing identical drop in the central
diffraction intensity.
Another
question that can be answered using diffraction calculation is what is
the difference in optical quality between long-focus achromats and
apochromatic refractor. It is known that "true" apos have only about 1/10
of the secondary spectrum of a comparable achromat, or less, but
this fact alone can't be used as the basis for a direct comparison. The
reason is that the primary source of chromatic error in a typical apochromat is not secondary spectrum, but sphero-chromatism,
which is in turn entirely negligible in long-focus achromats. Long-focus
achromat aficionados tend to place it very close to the "true apo" level optically, but the MTF
confirms that the latter does have noticeable advantage.
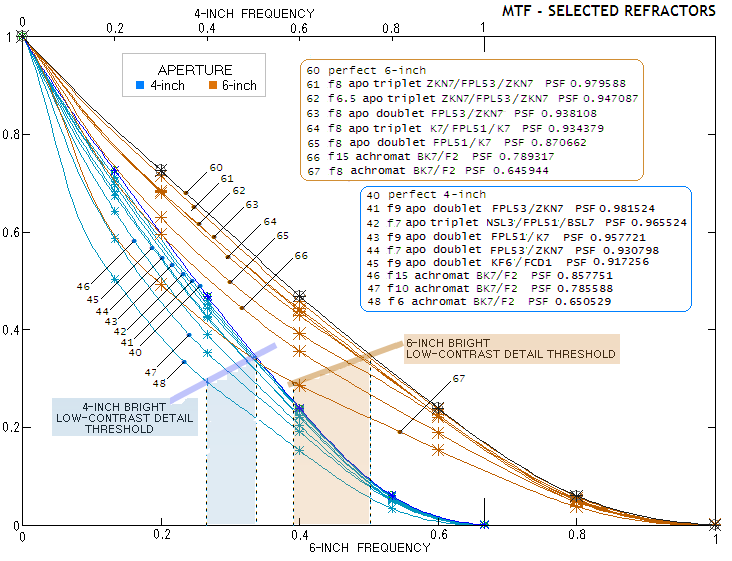
FIGURE 74: Polychromatic (photopic eye sensitivity) MTF plots for
selected 4 and 6-inch refracting objectives. A 6-inch triplet apo is
well into the "true apo" zone (above 0.95 polychromatic Strehl) at f/8,
and a tad short of it at f/6.5 with the best FPL53 match, Schott's ZKN7.
An f/8 doublet with these glasses is nearly as good as the f/6.5 triplet
and, for all practical purposes, identical to a second-class f/8 triplet
with FPL51 and one of its best matches, K7. An f/8 doublet with these
glasses, however, drops below 0.9 Strehl, positioned closer to
long-focus achromats than the true apos. With a 4-inch aperture, a
first-class doublet at f/9 comes as close to perfection as it may
matter, and the second-class triplet is nearly as good
at f/7. A second-class doublet can still be a true apo at f/9, but not
the first-class doublet at f/7. It is only slightly better - most likely
below the threshold of perception - than a second-class doublet at f/9,
in this particular case the old Meade f/9 apo. An f/15 achromat is about
as much behind them as they are behind near-perfect aperture. About as
much behind the f/15 is f/10 achromat, and the f/6 is nearly as much
behind the f/10, as the latter is behind the true apo.
A few notes: (1) all doublets are air-spaced, and all triplets are
oil-type, i.e. with lenses in contact and R2=R3 and R4=R5
(2) MTF plots are based on the design optimum, which somewhat favors the
apos, which generally have much higher sensitivity to misalignment, (3)
photopic Strehl is different than mesopic Strehl, which is lower and
generally applies to night time observing, and
(4) the plots do not include seeing error, which means that the gap
between the 6-inch and 4-inch apertures is - all else unchanged -
somewhat smaller under the real sky.
Above plots are polychromatic MTFs (440-680nm) for
the standard Fraunhofer doublet achromats, and several apochromats, for
4 (blue) and 6-inch apertures (pink). The difference in contrast level and resolution
between long focus achromats and apochromats is smaller for the 4-inchers,
as expected, considering that larger achromats have more chromatism for
given focal ratio. Long-focus 4-inch achromat is close to the faster
4-inch apos, but an f/10 apo has
near zero chromatism. Polychromatic Strehl values given for achromats
are those at the best (diffraction) focus (SP),
and their MTF plots are also for this focus location.
Note that these plots reflect only the level of
chromatic correction. Longer-focus systems have the additional advantage
of more relaxed fabrication and alignment tolerances, and can be
expected to have generally somewhat better correction level than what
the chromatic correction alone implies. Smaller apertures, of course,
are less subjected to seeing error, hence the difference in contrast
transfer is also somewhat less than what above plots imply.
The overall perception is probably that long-focus refractors should
have somewhat better optical rating than what approximation in (a)
indicates. Part of this notion results from the general tendency of assigning to
the high-quality performers better
optical quality than they really have. In other words, empirical
criteria is based on the performance relative to other instruments; a
telescope operating at a 0.9 Strehl overall optical quality, or even
less, will be perceived as being close to perfection if other telescopes
are operating at lower to significantly lower levels of optical quality
- which is commonly the case.
In this context, the perception of higher than actual optical quality
level springs from a number of error sources that are invariably present
being neglected or
downplayed. For instance, a superb 6" Maksutov-Cassegrain telescope that
goes with 0.96 Strehl optics will be operating far below that level in
the field. In the average 2 arc seconds seeing, average seeing-induced
error is around 0.1 wave RMS, enough to keep it below 0.7 Strehl level
half of the time, or so. It is not much better inside the tube: typical ~0.35D
central obstruction alone lowers the 0.96 optics Strehl by a 0.77
degradation factor, to 0.74. Thermally induced errors are all but likely
to push it further down, below 0.7 Strehl level. Combine it with the
seeing error, and you have an instrument performing not much above 0.5
Strehl most of the time (misalignment error is not as much significant with
Maksutov-type telescopes, in general, as it can be with some others). Yet it is regarded as a very good
overall performer. Its actual optical quality level? According to the
generally accepted scale shown on Fig. 73, it belongs to
"poor" optics.
Considering this, the 0.74 peak diffraction intensity resulting from
secondary spectrum in a 6" f/15 achromat -
or an effective ~0.77 for the range of resolvable extended details -
doesn't look bad anymore. While it still suffers the same from seeing,
it is significantly less affected by thermal errors, and - has no
aperture obstruction. Since its chromatic
error nearly offsets the effect of Mak's central obstruction, it is
likely to perform better in the field.
Combined with the approximate ratio of the
increase in "effective Strehl" for the left side of MTF graph, due to
the better contrast transfer resulting from higher relative encircled energy
within first maxima, given by
(F+10)/(F+9), best polychromatic Strehl of an achromat is approximated by
Sext~(F+10)(F+7)Spe/(F+9)(F+6),
or
S ext~ (F+10)Sp/(F+9)
(c)
This "effective Strehl" value better
reflects achromat's performance level for details about Airy disc in
size, and larger, including nearly all resolvable planetary details.
In conclusion, in order to assess level of performance of an achromat,
and to compare the effect of secondary spectrum to that of central
obstruction and spherical aberration, we have to depart from nominal
secondary spectrum, and turn to diffraction calculation. In addition,
the effects specified above - higher relative energy within 1st maxima,
and shift to best polychromatic focus - need to be taken into
account.
Based on these factors, following table shows the peak polychromatic Strehl
SP
(for the entire range of MTF frequencies) and the maximum effective
polychromatic Strehl
Sext
for the left side of MTF graph (extended details)
of an achromat with near-zero aberration in the optimized wavelength, for selected systems, with the corresponding value
of primary spherical aberration, as well as central obstruction size
(not adjusted for the effect of brighter central disk). The achromats
are standard, BK7/F2 , with the wavefront error in F and
C nearly equalized (this
secondary
spectrum mode gives somewhat higher polychromatic Strehl than the
canonical mode with F and C focus nearly coinciding). The
apo is an FPL52/ZKN7 doublet.
|
Refractor |
SP |
Sext |
Comparable
spherical aberration
(P-V) |
Comparable
central obstruction. |
|
SP |
Sext |
SP |
Sext |
|
4" f/5
achromat |
0.63 |
0.67 |
1/2.8 |
1/3 |
0.45D |
0.43D |
|
4" f/10
achromat |
0.79 |
0.83 |
1/3.9 |
1/4.4 |
0.33D |
0.30D |
|
4" f/15
achromat |
0.855 |
0.89 |
1/4.8 |
1/5.5 |
0.27D |
0.24D |
|
4" f/20
achromat |
0.90 |
0.93 |
1/5.8 |
1/7 |
0.23D |
0.19D |
|
6" f/15
achromat |
0.79 |
0.82 |
1/3.9 |
1/4.2 |
0.33D |
0.31D |
|
8" f/10
achromat |
0.64 |
0.67 |
1/2.8 |
1/3 |
0.45D |
0.43D |
|
4" f/10 apochromat |
0.99 |
0.99 |
1/18.9 |
1/18.9 |
0.07D |
0.07D |
|
36" f/19 (Lick refractor) |
0.49 |
0.52 |
1/2.2 |
1/2.3 |
0.55D |
0.53D |
TABLE 7:
Approximate comparative effects of secondary spectrum (according to
OSLO output) vs. spherical aberration and central obstruction. Comparable mid-to-low
frequency P-V error of spherical aberration is obtained substituting the
EE value for the peak intensity value (Sext)) in W~0.8√-logS,
to better reflect the effect of the out-of-disc energy, which is
generally lower for achromats than what the peak diffraction intensity
indicates. The comparable relative central obstruction size
ο is obtained from
Sext=(1-ο2)2,
thus ο=[1-Sext0.5]0.5. Note that this actually compares it to the encircled energy of the obstructed aperture, not its relative peak intensity, and does not take into account its reduced central maxima diameter.
Considering common perceptions among amateurs, the numbers for achromats may look a bit optimistic, but that is what
raytracing results imply. One likely exception is the Lick refractor
which, due to its enormous size, cannot have overall optical quality
compared to that of small amateur instruments based on the Strehl alone.
Also, a first-class 4" apo can have better design polychromatic
Strehl than 0.99, up to 0.999 for a 100mm f/10 system - at least in
theory. However, in addition to fabrication imperfections lowering
the Strehl value, common doublet
designs are extremely sensitive to even slight deviations from design
parameters; induced spherical aberration, even when only moderate, can
significantly lower their polychromatic Strehl. It may not be readily
apparent, since the chromatism in an apochromat is visually less
noticeable as a color error, for given error level (i.e. polychromatic
Strehl), due to non-optimized colors being tied together. But it does
lower contrast just the same.
Note that triplet apos are much more forgiving in that respect than
doublets, and that is their main practical advantage.
Polychromatic Strehl vs. chromatic Strehl
It is important to emphasize that polychromatic Strehl is not
necessarily an accurate indicator of chromatic correction. This
is because it includes optimized wavelength (and wavelengths close
to it). The less well corrected optimized wavelength, the lower is
polychromatic Strehl, even if the chromatic correction level remains
unchanged. And the other way around, any given polychromatic
Strehl value can result from a different level of chromatic
correction. For istance, 0.95 poly-Strehl with 0.96 central line
Strehl indicates Strehl degradation factor due to chromatism of
95/96=0.99. But if the central line Strehl is 0.99, the chromatism
degradation factor is 95/99=0.96. While the traditional long
focus achromats should have 0.99+ central line Strehl, fast
achromats and, more so, apochromatic doublets, can have the
limit to central line correction significantly lower, due to
their much tighter tolerances or, in the case of apochromats, the
higher-order spherical aberration residual as well.
It is also important to emphasize that all the above figures are valid for
photopic eye sensitivity and the standard e-line optimized achromat (C-F
correction). As we all know, most observing is done during night time,
when eye sensitivity shifts from photopic (bright light conditions)
toward partial dark adaptation (mesopic mode).
It is the most complex eye mode, with both cone and rod photoreceptors
simultaneously active. Cone sensitivity in the mesopic mode increases
for all wavelengths, but more in the blue and red than in green/yellow.
However, this expanded cone sensitivity is followed with the decrease in
their acuity. Even more so, this is the case with the rods, which are
much more sensitive in the blue/violet than the cones, but also having
much lower resolution and contrast sensitivity. At present, there is
probably not enough specific knowledge about eye's mesopic function to
assess level of optical quality in this mode. In general, it is to expect
that the increased sensitivity beyond photopic range, particularly
toward blue/violet, effectively increases polychromatic error in both
achromats and apochromats. This increase, however, is probably mainly offset by the lowered eye acuity.
Due to chromatic defocus in achromats being significantly larger toward
blue/violet end of the spectrum, they may be somewhat more affected than
apochromats, but it remains speculative until mesopic error level
- i.e. nominal contrast and resolution limits imposed by chromatism - can be evaluated versus specific contrast/resolution abilities of the mesopic eye.
◄
4.7.2. Lateral color error
▐
4.8. Fabrication errors
►
Home
|
Comments |
