telescopeѲptics.net   ▪   ▪   ▪  ▪ ▪▪▪▪ ▪ ▪  ▪   ▪    ▪     ▪      ▪       ▪        ▪         CONTENTS
 

◄ 6.6. Effects of aberrations: MTF  ▐    6.7. Coherent transfer function, Fourier transform ►
 

6.6.1. Aberration compounding and MTF, CCD contrast transfer, MTF limitations

Expressing combined effect of two or more aberrations through the MTF can be done, similarly as for the wavefront aberrations, either precisely, through a complex calculation, or as a single number extracted from statistically averaged probability (approximation). As the above graphs show, difference in the effect on performance of various aberrations with a similar RMS wavefront error level becomes more significant as the nominal error increases. For two or more aberrations combined, the effect is, on average, greater than either single aberration components alone. However, they do not add up arithmetically. For relatively small, unrelated or random wavefront errors, the combined effect is given by the square root of the individual errors squared, or

ωc= (Σωi2)1/2          (58)

with ωi being the individual RMS wavefront error. Similarly, the combined effect on peak intensity of the PSF (Strehl) and, thus, average MTF contrast loss can be, for mostly unrelated smaller aberrations, closely approximated as the product of individual Strehl ratios for any number i of different aberrations, Strehl being, in fact, an MTF (contrast) degradation factor:

Sc= S1S2 ... Si          (58.1)

The product  Sc is a combined Strehl (the peak diffraction intensity ratio) for the errors included; it also expresses the average MTF contrast level in units of the average contrast level of a perfect aperture. This implies that for this type of aberrations, the combined MTF as a product of the two aberrated MTF - i.e. their contrast transfers at every spatial frequency.

The conventional minimum acceptable level of optical quality - so called diffraction-limited standard - is 1/4 wave P-V wavefront error of spherical aberration or, in more universal form, the associated 1/13.4 wave RMS (0.80 Strehl). That corresponds to 0.42 wave P-V of coma, 0.37 wave P-V of astigmatism, and so on. This criterion is not strict - it is a somewhat lose dividing line between good and bad optics. In fact, since it is, in the commercial environment, habitually applied to the quality level inherent to optical surfaces alone, it is safe to assume that the actual quality minimum is lower, perhaps significantly. In addition to induced aberrations considered earlier, as well as chromatism, one of the factors lowering optical quality in addition to that determined by the wavefront aberration level alone is the effect of central obstruction.

Beside wavefront aberrations and pupil obstructions, contrast degradation can be caused by detector properties. Specifically, contrast transfer of a CCD chip depends on its pixel size, relative to the system Airy disc diameter. Contrast transfer in the final image is a product of contrast transfers of a telescope and that of the CCD chip. According to Schroeder (Astronomical Optics, p309), for square pixel with the side p in units of λF, contrast transfer (MTF) is given by:

with ct being the telescope MTF, the sinc term the MTF of CCD chip (the angle pνπ is in radians, converting to degrees in the numerator) and ν the normalized spatial frequency (for ν=0 cf is undefined, but it approaches 1 as cf approaches 0, thus represents its limit value).

     Sinc values for selected pixel sizes are plotted to the left. For p=2, which for an ƒ/5 system and λ=0.5μ corresponds to 5μ pixel size, contrast transfer drops to zero at ν=0.5 (from ct=sinπ/π=sin180°/π), effectively halving the cutoff frequency of a telescope before contrast reversal (negative modulation values). In addition, contrast level over all frequencies before reversal is degraded to the level of a twice smaller perfect aperture, with the contrast after reversal being a small fraction of the (original) contrast in a perfect aperture (FIG. 102). Half as large pixel preserves more of contrast transfer efficiency, but in order for it to approach that of a telescope - assuming its optical quality is not significantly below the usual standards - pixel size needs to be reduced to 0.5λF, or 1/2 of the system's FWHM. According to the Nyquist criterion, this is also the minimum needed for clearly resolved point sources at the diffraction limit ~λ/F.

     Since the first zero for the sinc factor falls at pν=1, first zero of the combined MTF is at the spatial frequency ν=1/p, regardless of the MTF of a telescope, as plots for p=3 and p=4 show. Hence, preserving the limiting MTF resolution of a telescope requires p=1, or smaller. Some positive contrast recovery at high frequencies does occur for p>2, but it is generally insignificant vs. the overall contrast/resolution loss.

FIGURE 102: Additional contrast degradation in 42% obstructed system resulting from relative pixel size p, in units of system's aberration-free FWHM (~λF). The combined system+detector MTF is a product of the system's and pixel's MTF (i.e. product of their respective contrast transfers at each frequency). For p=2, contrast drops to zero at spatial frequency ν=0.5; it still retains low negative, or reverse contrast (the bright lines become dark, and vice versa). For an f/8 imaging system and no significant aberrations present, this level of contrast loss would correspond to 8.8μ pixel size, for λ=0.55m. Smaller pixels deliver better contrast transfer, and are not prone to the occurrences of  the "negative" effect in the high-frequency range. To nearly preserve contrast transfer of the system in this case, pixel size shouldn't exceed 2.2μ. This requirement is significantly more relaxed in the average field conditions, mainly due to the actual FWHM at the detector being larger up to several times (depending on the aperture size and length of exposure) than the aberration-free FWHM's, as a result of blurring caused by atmospheric turbulence.

At this pixel size, the sinc factor for ν=0.5 (approximate MTF cutoff frequency for extended bright low-contrast details for aberration-free aperture), is sin45/(π/4)=0.9, degrading telescope's contrast transfer at this frequency by only 10%. Relative contrast degradation at this pixel size does increase for higher frequencies, which will also slightly reduce the point-source cutoff frequency.

Obviously, aberration level substantially degrading the actual contrast transfer of a telescope would correspondingly ease the limit to the maximum pixel size needed to nearly maintain this contrast transfer level. By significantly enlarging telescope's FWHM at the detector, the ever present seeing error puts the actual pixel size limit significantly above the formal Nyquist criterion level, for all but very small apertures. For short- and long-exposure imaging, in order to determine the approximate limit to the pixel size which nearly preserves contrast transfer efficiency of a telescope, the λF unit in the Nyquist criterion needs to be replaced with the respective seeing FWHM.

The effect of aberrations on CCD imaging can be also assessed in the context of ensquared energy.

Of course, other factors influencing CCD contrast transfer efficiency also need to be considered, particularly with respect to signal-to-noise (SNR) ratio, which can significantly affect contrast transfer and overall detection capability of imaging system. In general, the SNR ratio can be expressed as

SNR=SQt/√SQt+N,

with S being the signal flux from the object, Q the detector's quantum efficiency (defined as the ratio of detected vs. incident photons), t the exposure time and N the sum of all noise contributions, N=(BQ+D)t+R2, where B is the background (sky) emission, D the detector's dark count (thermally produced electrons within CCD chip itself) and R the readout noise.

Needless to say, presence of aberrations, either inherent to imaging system or induced, further reduces CCD contrast transfer efficiency and limits to resolution.  Ensquared energy, a quantitative indicator of the imaging quality shows how much of the total energy of a point image remains in a square of a given size, square center coinciding with the Gaussian focus. If chromatic errors are present, they will affect CCD imaging efficiency significantly more than visual image, due to the much higher sensitivity of the CCD chip to wavelengths toward the ends of the visual spectrum (note that all three plots, for the relative CCD sensitivity, photopic and scotopic eye sensitivity, are normalized to unit for their respective peak sensitivity).

A few words about limitations of the standardized MTF in projecting its output to the actual observing. One comes from the standardized MTF being strictly exact only for the object used: parallel, contrasty, brightly illuminated dark and bright lines with sinusoidal intensity distribution. For different types of objects, both contrast transfer and cutoff frequency (limiting resolution) may be different, possibly significantly. Also, the MTF assumes continuous spread of energy; in other words, every line interacts with all the energy reaching it from both, left and right. Actual observing objects are finite, and the absence of continuous spread results in the outer areas being subjected to less of energy overlapping than the MTF assumes. It is particularly pronounced with small, or narrow objects (FIG. 103).


FIGURE 103: LEFT: Typical continuous MTF pattern exposes every single point to all the energy from all surrounding points that extends to it (gray circles). All bright lines between A and B will contribute to the energy spread out by diffraction and aberrations to the mid point marked by the cross. MIDDLE: With relatively small ( with regard to the diffracted/aberrated energy spread radius) object image, significant portion of the energy lost to the Airy disc will spread out of object area (C), with comparatively less energy affecting the image itself. It would result in better contrast transfer than what MTF indicates for given aberration level.
RIGHT: Example of the effect of pattern extent on contrast transfer. Image of a 3-bar square wave pattern has higher contrast transfer at all frequencies than continuous pattern, as well as somewhat better limiting resolution (cutoff frequency); the effect is similar with 3-bar sinusoidal pattern (standard MTF).

The consequence is better contrast transfer and resolution than what the MTF (thus, also PSF and the Strehl) would indicate, even for an object identical to the standardized MTF pattern. Specifics of this effect vary with the object type, as well as the type and level of aberrations present.

Finally, a limitation of the MTF - but not OTF - is, as mentioned on the previous page, that it does not include information on the phase shift and its possible effect on the image. Phase shift itself merely shifts the MTF diffraction image laterally with respect to the Gaussian (geometric) image, and shift of half the phase (π radians) will result in contrast reversal, i.e. switch of light areas into dark, and vice versa. While neither is affecting the definition of MTF pattern, which is evaluated at each isolated frequency alone, an actual image will often contain multiple frequencies, either isolated or in continuums. In such case, intensity distribution shifts caused by phase shift can result in deformation of image vs. object, which could also impair detail detection or resolution. Some examples of this effect are given below. One of the objects used is the continuum of frequencies contained in the MTF (aberration-free) resolving range, and the other a radial test pattern (simulations generated by Aberrator; aberrated images were made more contrasty than the original output, to make the effects on patterns more obvious).

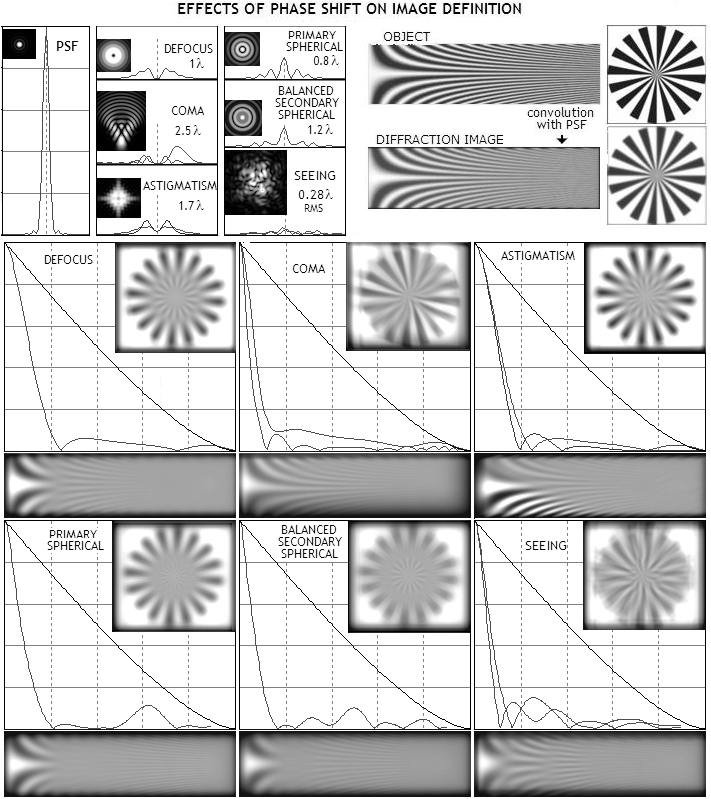
    Aberration-free and aberrated PSFs are shown top left (the plots are not proportional to the simulations scale-wise, but either are among themselves, double PSF/MTF plots for asymmetrical aberrations are for the best and worst PSF orientation). Magnitude of aberrations are chosen to roughly produce the contrast transfer level corresponding to 1λ wave P-V of defocus (with this large aberrations even the RMS error is not a reliable indicator of error magnitude, and so is not the PSF central intensity). Asymmetric aberrations, coma and astigmatism are given at a rotation angle midway between their best and worst orientation (indicated as two colored plots on their PSF and MTF graph) - 45 and 22.5 degrees respectively (counterclockwise) - for which the effect should be at least roughly averaged up.

    Defocus causes contrast reversal, changing the appearance of both images. Coma causes obvious asymmetry. Astigmatism causes an interesting effect that appears as contrast reversal in the star pattern, but only as intensity shift in the frequency continuum pattern (the difference in appearance for the lower and upper left portion of the image is caused by the diffraction pattern, rotated 22.5 counterclockwise, being nearly aligned with its "best" axis with the former, and with its "worst" axis with the latter). Primary spherical has lower contrast transfer in low and lower-mid frequencies, compensated by higher transfer in the high-mid frequencies, but the only change in image appearance is due to the insufficient contrast at some frequencies. Balanced secondary spherical, even if generally similar to the primary spherical, and at a roughly similar magnitude level, causes noticeably different effects. And the effect of seeing is in some ways similar to coma having, due to the extent of PSF energy spread, lower an early contrast drop and, due to its asymmetry, detail deformations. However, unlike coma it causes quite pronounced contrast reversals.

    None of these effects that can significantly lower image quality and detection level is indicated by the MTF. exception being, of course, low contrast level in the case of spherical aberration (spherical aberration also causes contrast reversal at the roughly doubled error magnitude). While this may not be significant in general terms, since this error magnitude is significantly larger than the average found near axis in amateur telescopes, farther off axis this level can be easily met and exceeded, thus effects of this type are the reality. Also, larger amateur telescopes routinely operate at these and larger error levels due to seeing, hence these kind of effects are no stranger there, even on axis.
 

◄ 6.6. Effects of aberrations: MTF   ▐    6.7. Coherent transfer function, Fourier transform ►

Home  |  Comments